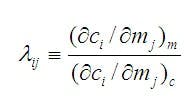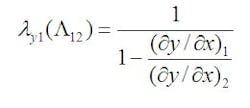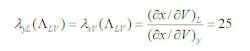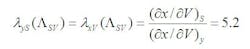Multivariable Control of Distillation
The fisrt part of this article was printed in CONTROL's May 2009 edition. Part two is included in June 2009 and part three in July 2009. Below is the complete article.
By F. Greg Shinskey
For the last two decades, model predictive control (MPC) has been promoted for multivariable processes, particularly in petroleum refineries. Yet its performance hardly justifies its promises, and economic payout is spotty at best. A. G. Kern in his article, “Outlook for Multivariable Predictive Control,” in the October 2008 issue of Hydrocarbon Processing cites “lackluster performance” of MPCs. In the March 2006 issue of the same magazine, Y. Z. Friedman in “Where Has Engineering Judgment Gone?” suggests that these “applications have not been successful.” This author is currently involved with a major refinery, proceeding from one distillation column to the next, replacing disappointing MPC systems with structured advanced regulatory controls (ARC), achieving substantial economic benefit and operator acceptance. The purpose of this article is to analyze some of the factors limiting MPC performance and demonstrate how ARC systems overcome them.
Quality Control
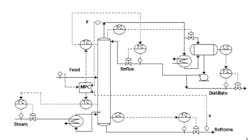
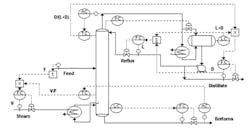
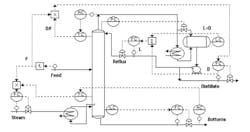
Most refineries require quality control over both top and bottom products to minimize energy consumed in separating their components. Product compositions are historically inferred from temperatures a few trays removed from where the product is withdrawn. This location is selected to minimize influence from off-key components and to increase sensitivity to manipulated variables. Ultimately, product compositions are usually measured in an on-line analyzer. The analyzer may have a sample interval of 10 minutes or more, along with sampling-line delay and lags associated with capacity in the reflux drum and column base. The temperature measurements—although less accurate—are far more responsive than the analyzers, both to disturbances and to manipulated flows. As a result, it’s common practice to control compositions by adjusting temperature set points in cascade, either using PID controllers or with a multivariable MPC (Figure 1). This structure has been published in handbooks and papers over the years, and can be considered standard practice.
As shown, the typical column has five pairs of manipulated and controlled variables―two product flows, reflux, reboiler heat and condenser cooling―used to control two levels, pressure and two temperatures. Analyzers report the overhead vapor and bottom compositions, y and x respectively. Condenser cooling is most often used to control pressure, in this example by partially flooding the condenser with condensate by means of the hot-vapor-bypass valve. The assignment of level controllers to manipulate the flow of their respective products is most common, leaving temperatures to be controlled by reflux and boilup. Disturbance variables are feed rate, composition and enthalpy, and coolant temperature.
The structure represented by Figure 1 just happens to be the least controllable of all possible single-loop configurations, as well as the most obvious. The principal difficulty is that the manipulation of reflux and boilup has almost the same effect on both temperatures. In other words, increasing steam flow will raise the top temperature almost as much as it raises the bottom temperature, and increasing reflux flow will lower both by similar amounts. In most columns, these temperature loops are therefore tightly coupled, and no amount of clever set point adjustment by the MPC can break through that tight coupling. The reflux-boilup control structure is marginally stable, presenting the MPC with what is known as an ill-conditioned matrix (See A. Hugo’s “Limitations of Model Predictive Controllers,” in the January 2000 issue of Hydrocarbon Processing.) The result is a tendency for the two temperature controllers to cycle against one another. While multivariable MPC has decoupling built into it, the coupling here is between the inner temperature loops, and the MPC is unable to defeat it from the outside.
Loop Interaction in Distillation
While there are five manipulated and controlled variables, their interaction exists in two distinct dynamic domains. For example, changing reflux flow causes the level in the reflux drum to begin moving immediately, while the top temperature may take several minutes before responding at all. The period of oscillation of a typical temperature loop on a column is about 10 to 40 minutes, while that of the level and pressure loops is more like one to two minutes. As a result, imbalances in the vapor and liquid traffic in the column appear as variations in liquid levels and pressure long before they affect temperatures, and these effects can be used to detect and counteract disturbances.
Being slower, the temperature loops can’t upset levels or pressure significantly, so interaction in this direction is minimal. The principal interaction problem exists between the two slow temperature (composition) loops. Their very similar dynamics makes each especially sensitive to oscillations developing in the other loop at the same period—a resonant effect. The two different sets of dynamics allow interaction between the temperature loops to be analyzed alone as a 2x2 system, assuming level and pressure loops closed as necessary.
The severity of the temperature-loop interaction varies greatly from one column to another, but may be estimated by comparing the relationship between the two product compositions as we hold a particular manipulated variable (MV) constant. The ideal case of regulation is where holding a particular MV constant results in one of the product compositions remaining nearly constant regardless of changes in the other MVs. This is not really achievable, so we are left to choose the best of the available MVs for regulation of the two compositions.
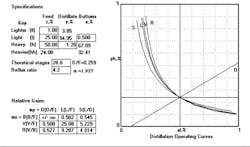
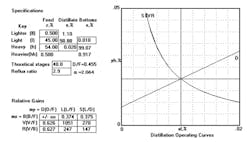
Figure 2 presents the choices available for a typical refinery column as determined by a four-component model. An example of a set of specifications appears in upper left corner. The feed is characterized as consisting of four components: light key (l) and lighter (ll), and heavy key (h) and heavier (hh). The split occurs between light and heavy key components; all the ll-key components exit overhead, and all the hh-key components leave the bottom. (There may be any number of actual components, but this model requires lumping them into these four groups.) Data representing desired operating conditions are entered into the blocks, and all the remaining values are then calculated by the model. The last item calculated is the relative volatility (α of the key components, which can be compared against its handbook value to test the validity of the model.
From this information, the operating curves are plotted, which cross at the specified product-composition coordinates. The curves relate the two controlled compositions in terms of key impurities: yh is the controlled heavy-key component in the distillate, and xl is the controlled light-key component in the bottoms. There are five independent MVs available to control the compositions, appearing left-to-right in the plot—all are treated as ratios by the model:
- Separation S, a function of the reflux/distillate (L/D) ratio
- Reflux L, actually reflux/feed (L/F) ratio
- Boilup V, actually boilup/feed (V/F) ratio
- Boilup/bottoms ratio R = V/B
- Distillate D, actually distillate/feed (D/F) ratio; bottoms B is dependent.
Each curve represents the x-y relationship when a selected MV is held constant while any other is varied. If two MVs produce the same curve, they are not independent—note that the L and V curves are almost identical.
Relative Gain Analysis
The magnitude of interaction between the composition (temperature) loops can be numerically estimated as the relative gain of any pair of MVs. RG is defined as the ratio of the open-loop gain with the other loop open to its value with the other loop closed. It can be expressed mathematically as the partial derivative of any controlled variable, ci, with respect to any manipulated variable, mj, with the other m held constant to that partial derivative evaluated with the other c held constant:
The RG λij is a dimensionless number with very definite properties. A value of 1.0 indicates no or only one-way interaction and is the best outcome. Positive values above 1.0 describe increasing levels of interaction caused by positive feedback through the other loop. Negative values indicate dominant positive feedback and must be avoided. Values approaching zero also indicate increasing interaction through negative feedback. Values in the range from 0.5-10 have the best prospects for control.
The relative gain values for a distillation column are readily calculated from the slopes of any two curves (1, 2) as they cross the operating point, where subscripts 1 and 2 represent the MVs selected to control y and x respectively:
The table in the lower left of Figure 2 gives RG values calculated from the operating curves for the example column. The three candidate MVs (my) for top composition control are S, L, and D, and the three (mx) for bottom composition are B, V, and R. Since D and B are mutually dependent, the relative gain for that combination is listed as ± ∞. As expected, the RG for the L-V combination is highest at 25.
A multivariable MPC is capable of decoupling if used in place of the TCs in Figure 1, instead of outside of them as shown. However, the higher the relative gain, the more sensitive the system is to error in the decoupler. For a structure having a relative gain of 25, a decoupler error (model mismatch) of as little as 2% can drive the decoupled relative gain from 1.0 to infinity (Shinskey, F. G., Process Control Systems, 4th ed., McGraw-Hill, New York, ’96, p. 269). In other words, a system having high RGs is not only difficult, but dangerous to decouple.
Restructuring
Fortunately, there is a way around the problem: restructuring the lower tier of control loops. There is no mandate that the product flows be under level control or that the temperature controllers manipulate reflux and boilup. Referring to Figure 2, we see that the RG can be dropped from 25 to 5.2 by manipulating reflux ratio instead of reflux flow to control top composition (temperature). A structure that accomplishes this is shown in Figure 3.
Reflux ratio is actually L/D, but holding any function of L/D constant will produce the S operating curve. In Figure 3, the top TC manipulates D/(L + D), which is 1/(1 + L/D). The level controller in the reflux drum manipulates the sum of reflux and distillate flows (L + D), and responds to a change in boilup V by moving them both at the same time while retaining their ratio intact.
Feed rate is used to set steam flow in ratio to it through a feed-forward loop. Following a change in feed rate, the dynamic compensator [t] lags and delays the flow signal, simulating the dynamics in the lower half of the column. Then the steam flow responds in ratio to the feed rate at the time the new feed rate reaches the column base. The resulting change in vapor flow travels up the column, is condensed, and raises the level in the reflux drum. The level controller then changes both L and D, maintaining the current ratio.
Economic Benefits
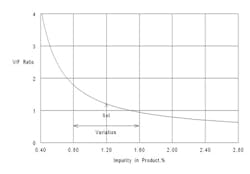
The lower relative gain of this structure has two benefits: less cycling and tighter control. Cycling brings with it an economic penalty associated with the nonlinear relationship between energy consumption and either product composition (Figure 4).
When a PID controller is cycling continuously, its integrated error will tend to be zero, so that the average product composition over time will be equal to the set point. However, due to the nonlinear relationship shown in Figure 4, the average level of energy consumed is higher than that required for a constant composition at that same set point in proportion to the amplitude of the cycle. For example, more energy is used to reduce the impurity to 0.8% than is saved at 1.6%—the cycling penalty.
The relative gain for a particular structure varies with the open-loop process gain, the numerator in Equation (1). For example,
Now if we change the structure of the top-composition loop, lowering the RG, the open-loop gain of the bottom-composition loop decreases by the same factor:
Dividing Equation (3) by Equation (4) gives a gain reduction in the bottom-composition loop by a factor of about five when the top loop is restructured. There is a similar reduction in that of the top loop.
This allows the proportional bands of the two temperature controllers to be reduced by the same factor of five, thereby reducing the deviation following disturbances by a factor of five—tighter control! This has been proven by field experience. Tighter control means that the set points for the composition (temperature) controllers can be moved that much closer to specification limits, resulting in further savings in energy.
There is one more economic factor that can be of major importance in refinery columns. They’re all limited in how much vapor traffic they can carry at the reboiler, at the condenser and in the column itself. Reducing the V/F ratio as described in Figure 4 allows the potential for increased feed rate F within the limits placed on V.
High-Purity Columns
With some columns, the high purity demanded of the products results in relative gains exceeding 100, such as shown in the example of Figure 5, which are typical of benzene and toluene columns. Note how all the operating curves collapse into one, with only the material-balance line separate. An RG of over 1,000 guarantees that the structure of Figure 1 will fail to control both product compositions. The only way to succeed here is to manipulate one of the product flows to control its own composition, called “material-balance control.” The relative gain of 0.626 recommends distillate flow to control distillate composition.
The structure used to accomplish this is shown in Figure 6. Feed flow is sent as a feed-forward signal to both composition loops, as all flows in the column must move in ratio to the feed rate. Moving distillate flow affects top temperature (composition) only indirectly, for the first result of any change appears in the liquid level in the reflux drum. Reflux flow must change by an amount equal and opposite to the change in distillate flow to force the external material balance on the internal vapor-liquid balance. If the overhead LC is left alone to adjust reflux flow in response to distillate flow, there will be a lag equal to the residence time of distillate in the drum, multiplied by the proportional band of the LC. This could amount to several minutes lag and substantially delay the top temperature loop. Dynamic response is even worse when separating close-boiling mixtures, like propane-propylene, where the temperature gradient is so small that its measurement isn’t useful for control, and the analyzer controllers have to respond to load changes.
The dynamic response of the top composition (temperature) loop is greatly enhanced by the simple decoupler shown as a summing block [∑] in Figure 6. It converts changes in distillate flow to opposite changes in reflux flow, eliminating any delay. The output of the LC then becomes (L + D), from which the measured flow D is subtracted to produce the set point L for reflux flow control.
It’s imperative that the material-balance loop remain closed all the time, for if it’s left in manual, then neither composition can be controlled. The withdrawal of distillate must match the amount of the light component entering in the feed, and this requires that the top composition (temperature) feedback loop be closed. No amount of adjustment to boilup can overcome an incorrect distillate flow.
Material-balance control has the added advantage of protecting the column against heat-balance upsets. Any change in heat input, feed enthalpy or reflux temperature is prevented from affecting the distillate flow, and therefore disturbing distillate composition. With an L-V structure, these disturbances are magnified by the L/D ratio and passed on to the material balance.
The Role of MPC
Having imposed the appropriate control structure on the column to provide tight temperature control, there’s little work for the analyzer controllers to do. An MPC applied at that level will probably perform satisfactorily and can be used for optimizing set points.
While MPCs are capable of constraint control, these need to be applied at the lowest level, rather than at the highest. For example, the column needs to be protected against flooding and overpressure—the former represents a column limit and the latter a condenser limit. The flooding limit is most reliably imposed by a column differential-pressure controller overriding steam flow through a low-signal selector at the steam valve; a second pressure controller can also override steam flow in the same way. This method of applying constraints is simple, reliable, dynamically effective, and proven over many, many years. Protection against integral winup is described in my May 2006 article in Control, “The Power of External-reset Feedback.”
In conclusion, as Y. Z. Friedman suggests, controlling multivariable processes is more than simply throwing the latest matrix-based MPC at them—sound engineering principles must also be applied. [end]