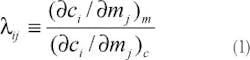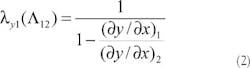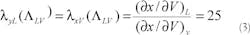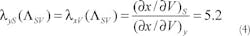Multivariable Control of Distillation, Part 2
This article was printed in CONTROL's June 2009 edition.
Editor’s Note: Part 3 of this series will appear in the July issue of Control.
By F. Greg Shinskey
The magnitude of interaction between the composition (temperature) loops can be numerically estimated as the relative gain of any pair of manipulated variables (MVs). RG is defined as the ratio of the open-loop gain with the other loop open to its value with the other loop closed. It can be expressed mathematically as the partial derivative of any controlled variable ci with respect to any manipulated variable mj with the other m held constant, to that partial derivative evaluated with the other c held constant:
The RG λij is a dimensionless number with very definite properties: A value of 1.0 indicates no or only one-way interaction and is the best outcome. Positive values above 1.0 describe increasing levels of interaction caused by positive feedback through the other loop; negative values indicate dominant positive feedback and must be avoided. Values approaching zero also indicate increasing interaction through negative feedback. Values in the range from 0.5-10 have the best prospects for control.
The relative gain values for a distillation column are readily calculated from the slopes of any two curves (1, 2) as they cross the operating point:
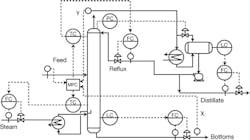
where subscripts 1 and 2 represent the MVs selected to control y and x respectively. The table to the right in Figure 1 gives RG values calculated from the operating curves for the example column. The three candidate MVs (my) for top composition control are S, L and D, and the three (mx) for bottom composition are B, V and R. Since D and B are mutually dependent, the relative gain for that combination is listed as ±∞. As expected, the RG for the L-V combination is highest at 25.
A multivariable MPC is capable of decoupling if used in place of the TCs in Figure 2, instead of outside of them as shown. However, the higher the relative gain, the more sensitive the system is to error in the decoupler. For a structure having a relative gain of 25, a decoupler error (model mismatch) of as little as 2% can drive the decoupled relative gain from 1.0 to infinity. In other words, a system having high RGs is not only difficult, but dangerous to decouple.
Restructuring
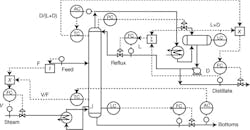
Fortunately, there is a way around the problem—restructuring the lower tier of control loops. There is no mandate that the product flows be under level control, or that the temperature controllers manipulate reflux and boilup. Referring to Figure 1, we see that the RG can be dropped from 25 to 5.2 by manipulating reflux ratio instead of reflux flow to control top composition (temperature). A structure that accomplishes this is shown in Figure 3.
Reflux ratio is actually L/D, but holding any function of L/D constant will produce the S operating curve. In Figure 3, the top TC manipulates D/(L + D), which is 1/(1 + L/D). The level controller in the reflux drum manipulates the sum of reflux and distillate flows (L + D), and responds to a change in boilup V by moving them both at the same time while retaining their ratio intact.
Feed rate is used to set steam flow in ratio to it through a feed-forward loop. Following a change in feed rate, the dynamic compensator [t] lags and delays the flow signal, simulating the dynamics in the lower half of the column. Then, the steam flow responds in ratio to the feed rate at the time the new feed rate reaches the column base. The resulting change in vapor flow travels up the column, is condensed and raises the level in the reflux drum. The level controller then changes both L and D, maintaining the current ratio.
Economic Benefits
The lower relative gain of this structure has two benefits: less cycling and tighter control. Cycling brings with it an economic penalty associated with the nonlinear relationship between energy consumption and either product composition shown in Figure 4.
When a PID controller is cycling continuously, its integrated error will tend to be zero, so that the average product composition over time will be equal to the setpoint. But due to the nonlinear relationship shown in Figure 4, the average level of energy consumed is higher than that required for a constant composition at that same setpoint in proportion to the amplitude of the cycle. For example, more energy is used to reduce the impurity to 0.8% than is saved at 1.6%—the cycling penalty.
The relative gain for a particular structure varies with the open-loop process gain, the numerator in Equation (1). For example,
Now if we change the structure of the top-composition loop, lowering the RG, the open-loop gain of the bottom-composition loop decreases by the same factor:
Dividing Equation (3) by Equation (4) gives a gain reduction in the bottom-composition loop by a factor of about 5 when the top loop is restructured. There is a similar reduction in that of the top loop.
This allows the proportional bands of the two temperature controllers to be reduced by the same factor of 5, thereby reducing the deviation following disturbances by a factor of 5—tighter control! This has been proven by field experience. Tighter control means that the setpoints for the composition (temperature) controllers can be moved that much closer to specification limits, resulting in further savings in energy.
There is one more economic factor that can be of major importance in refinery columns. They are all limited in how much vapor traffic they can carry at the reboiler, at the condenser and in the column itself. Reducing the V/F ratio as described in Figure 4 allows the potential for increased feed rate F within the limits placed on V.
Greg Shinskey is a process control consultant, an ISA Fellow, a member of the Process Automation Hall of Fame and the recipient of the ISA Lifetime Achievement Award.
Editor’s Note: Part 3 of this series will appear in the July issue of Control.