By Béla Lipták, PE, CONTROL Columnist
To most of us, the term "optimization" implies complexity. It suggests partial differential equations and multidimensional peak searching. It implies the stuff that is for egghead theoreticians and not for the down-to-earth engineers in real-world processing plants. In our plants, where pipes leak, sensors plug, and pumps cavitate, the role of the plant engineer is more like that of a fireman than that of a scientist.
Yet optimization is a friend of the plant engineer! It does not need to be esoteric or theoretical-it can provide practical solutions to real problems, it can maximize productivity while minimizing costs. And most importantly-because of its fully automated nature-it can actually free the plant engineer to fix those leaking pipes and pump seals.
Optimization is nature's way of control: A tree, for example, is simultaneously reacting to all the variables that affect it. Similarly, in a fully optimized plant, levels, temperatures, pressures, or flows should only be constraints. They should all be allowed to float within their predetermined safe limits while the efficiency or productivity of the operation is continuously maximized.
This is different from the earlier design philosophy, where levels and temperatures were held at constant values (if you keep a level constant, what is the tank for?) and unit productivity was an accidental and uncontrolled consequence of these rigidly held values.
Control Evelope
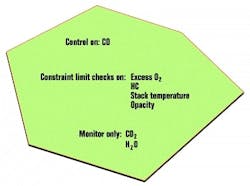
The key tool of optimization is the multivariable control envelope. This is a polygon with its sides representing the various constraints of the particular process. In case of a boiler, for example, one might simultaneously monitor excess oxygen, carbon monoxide, unburned hydrocarbons, stack temperature, and opacity, and assign the sides of the polygon to represent the allowable limits on each.
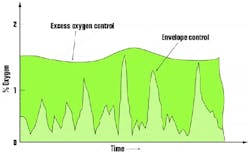
Whenever a limit is approached (for example, the CO limit in Figure 1 above), control is switched to that controller, but as soon as the process approaches another limit, control is transferred from the CO controller to that other variable. Through this multivariable control strategy, the boiler is "herded" to stay within the envelope and thereby a much faster and more sensitive control is provided (see Figure 2 below).
FIGURE 2: MORE SENSITIVE CONTROLAnother advantage of envelope control is that it provides an extra degree of freedom. This means that an additional controller can be activated when all constraints are within their limits (when the process is inside the control envelope). During these periods, we are free to manipulate the process by a new controller, which can maximize efficiency.
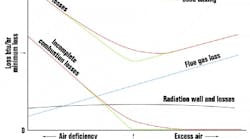
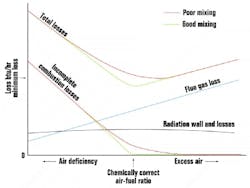
The efficiency of combustion would be at a maximum when every carbon atom found two oxygens to unite into a CO2 molecule. Under these ideal conditions, no unreacted excess oxygen would remain to leave with the flue gases. In real combustion, the mixing of fuel with air is never perfect. Therefore some air (including its 79% nitrogen) will always enter the combustion process at ambient temperature and will travel through the boiler "just for the ride," picking up valuable heat and then wasting that heat as it leaves with the flue gases.
In a combustion process, the losses are the sum of the radiation losses, the flue gas heat losses (through the stack), and the unburned fuel losses caused by incomplete combustion. For each boiler, the sum of these three loss curves (the total loss curve) has a minimum (see Figure 3 below), which identifies the point where efficiency is the maximum. When the boiler is operating inside its constraint envelope, the optimizer controller will automatically shift it towards this maximum-efficiency point.
FIGURE 3: CUTTING LOSESThe efficiency target for coal-fired boilers is 88-89%; for oil-fired, 85-87.5% ; and for gas-fired, 82-82.5%. An added benefit of such optimization strategies is that whenever the actual boiler efficiency drops below the above listed targets, the operator knows that it is time for maintenance.
Finding the Optimum
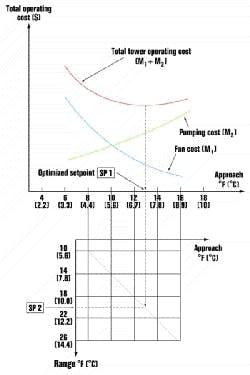
Just as the total loss curve of a combustion process has a minimum, so do most other processes. For example, the cost of meeting the cooling water needs of a plant can also be represented by a curve that has a minimum. Such a minimum point results whenever the total cost curve is the sum of two curves of opposite slopes.
In the case of the cooling water supply system, these are the pumping and fan cost curves. If the temperature of the plant cooling water supply is reduced (approach to wet-bulb lowered), the cost of cooling water pumping also drops because less of this colder water needs to be pumped, but the cost of cooling-tower fan operation is increased because more air flow is needed to generate the cooling water supply at this lower temperature.
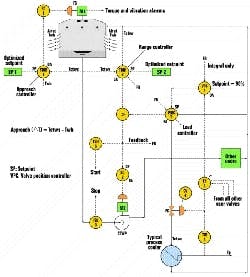
The total cost will optimized when the sum of the fan and pump station costs is minimized. The task of the optimizer controls (see Figure 4 below) is to bring the levels of fan and pump station operations to this optimum point. This is achieved by setting the approach (Tctws-Twb) setpoint on TDIC-1 to match the minimum point on the cost curve (see Figure 5 below).
FIGURE 4: OPTIMIZER CONTROLSThe Broader View
In the industrial plants of the 21st century, automatic optimization will become more common. While in the earlier examples I defined optimum as the operation at minimum cost, in fact optimization is much more flexible and can do much more than just provide maximum production at minimum cost. One can, for example, also optimize a plant to maximize its safety or its useful life, or to minimize the pollution it causes or the energy it consumes.
Therefore, using envelope optimization as a tool, the next generation of process control engineers will be able to convert today's technology (which is market-driven, amoral, and value-free) into a force that will not only turn a profit, but will also improve the quality of our lives.